Analysis of the problem

There are a number of persistent problems with numeracy in vocational education.

1. There is insufficient consensus on the form and content of numeracy based on what is useful and necessary for vocational students in this century.

2. Due to the recent policy history of top-down mandatory (exam) tests, there is a focus on thresholds and passing exams, rather than on developing practical and functional skills among students, in a way which keeps everyone on board.

3. The influx of students into vocational; education will become more diverse in the coming years, partly as a result of the abolition of the national numeracy increased irregular (young) adult inflow .

4. Many parties in and around vocational education think in terms of gaps instead of progress, in terms of remedies instead of development. This invites the repetition of the lesson material and teaching approaches from previous programmes, which both have demonstrably missed their goal in the past. It also strengthens the already widespread math anxiety among students.

5. From a national point of view, very little attention is paid to the numeracy skills that already exist among incoming students.

Possible solutions

Within vocational education,  a future-oriented vision on the development of numeracy is necessary. But the elaboration of such a vision in educational practice must also be consistent with the vision and must not fall back on old – usually poorly functioning – forms of education and testing.
By numeracy, we mean functional mathematics linked to situations in which mathematics is indispensable in order to solve real, practical problems, to form an opinion or to make a decision.
When developing such a vision for educational practice, the following points of attention are of importance.

1. When developing a vision, think in terms of literacy and numeracy. This is in line with the international concept of ‘literacies’, in which the required knowledge, skills and competencies are mainly seen as a ‘social practice’, strongly anchored in the daily and future actions in education, profession and society. This makes numeracy a self-evident factor in the preparation for professional practice and the rapidly changing professional context that will follow. But it is also a necessary skill for citizens and is important when connecting with (rapidly changing) further education and training. In doing so, make use of international developments relating to numeracy, as defined for example in the PIAAC Numeracy Assessment Framework (OECD) or in the Common European Numeracy Framework (under development).
2. Give a practical interpretation to the various functions that numeracy can have in the role of (future) professional practitioner, citizen and lifelong learner (in further education). Focus on functional mathematics , i.e. linked to situations in which mathematics  is indispensable for solving real, practical problems, forming an opinion or making a decision. Therefore, focus on the frequent solving of practical situations to be counted on, frequent interpretation of quantitative messages/information, regular decision-making based on quantitative data, the conceptual and adequate use of calculators and the critical consideration and use of apps, which take all kinds of calculations off your hand.
3. Consider numeracy as a competence that can best be developed, stimulated and accelerated with good educational activities within the “zone of proximal development”  of the student, such as the education and professional practice and/or experiences from the proximity of daily (school) life and internship. This promotes the insight that being numerical is useful (and necessary). In general: place functional mathematics in a context that makes students curious about a solution and prevent as much as possible aimless mathematics.
4. Recognize that psychological factors surrounding mathematics are just as important as cognitive factors. Think of widespread math anxiety, aversion and alienation that can occur among students as a result of education that merely focuses on right-wrong  feedback to the student or merely focuses on instruction.
5. Recognize that a number of facets that are conditional on the development of numeracy cannot be tested in a written or digital test. Examples are self-confidence, the ability to apply in more or less new situations, the ability to use numeracy skills in practical situations and communicating and presenting with the aid of numbers and data.
6. Concepts that should be avoided around mathematics and numeracy are: diagnosis, backlog, remediation, deficits, summative testing. These are all concepts which are too much related to fear of failure, fear of mathematics, backlog and feelings of inferiority. They do not in any way contribute to a student’s sense of purpose in order to develop his/her numeracy.
7. Assume that the influx of students into vocational education will always be and remain diverse, both in terms of level and educational needs. Leave the thought that all students can be served with one method and one exam. One size fits all does not exist in (vocational) education. More personalisation and more personal action plans for the acquisition of subskills (with possible sub-certificates) can be easily designed for numeracy education.The assumption that all students at vocational education at level 2, 3 or 4 will or should reach a certain generic level also does little justice to the reality within the vocational education student population. It is at odds with the desire to develop all students (inclusion) and to do justice to differences between students (diversity).
8. Of course, the foregoing does not rule out the possibility that demands can and should be made of students, but these will differ from one student to another. For successful mathematics education, requirements on students must be proportional and take into account the present prior knowledge, the present attitude towards mathematics, the chosen study programme and the chosen level within it. In order to achieve the objective of ‘numerical students’, attention must also be paid to offering a rich computing environment that is related to his/her zone of proximal development.

A practical solution: Working with Open Badges

The above is simpler said than done, but the development of Open Badges on Numeracy may offer a substantial part of the solution when it comes to programming, testing and certification.

Over the past ten years, we have begun to think in terms of Dutch reference levels 1F, 2F and 3F. A way of thinking that may or may not fit a student in a 1F, 2F or 3F box.  There are or were no middle ground.

If we open our minds to validated mini-certificates that not only make it possible to obtain from in junior general education, but that can also be adapted to profe4ssions and further education, then a whole new world will open up. Such a system is now technologically and practically available and free of charge, namely in the form of so-called Open Badges.

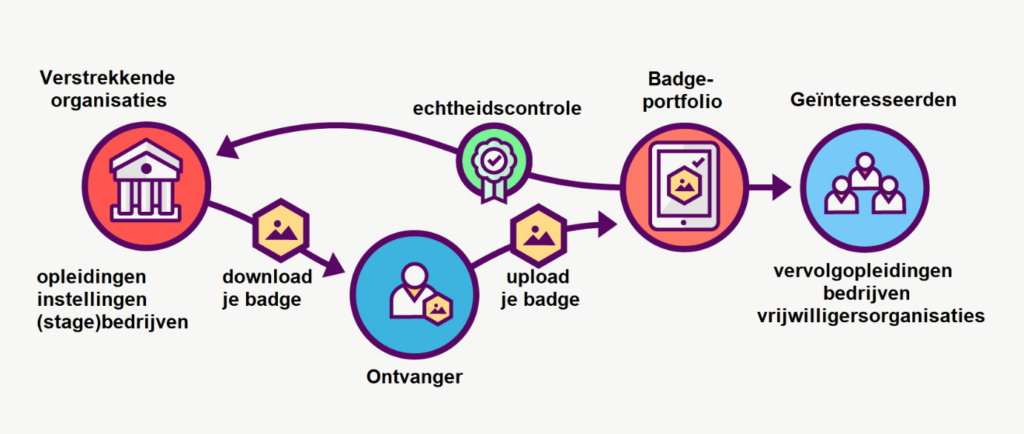
An Open Badge is a digital and validated certificate with which you can reliably prove that you are competent (have the right knowledge, skills and attitude) in a (small) part of a curriculum.

Open Badges can be developed in close relationship with the qualification files of programmes.
The wider and more frequent the use of Open Badges, the more they become a de facto standard.
The typical attributes of an open badge make it very suitable for basic functional skills, such as numeracy, namely: relatively small in size, free and open, transferable, stackable, evidence-based and usable in various environments.
Open Badges could therefore play an important role in mapping out the competencies of the intake at ROCs, which in turn could lead to targeted interventions to further develop the numeracy of individual students.

Agreements must of course be made with the supply secondary education schools in this regard. For examples, see Appendix 2.

Open Badges are a worldwide phenomenon.
In Europe, Europass makes use of Open Badges. Europass is an initiative of the European Commission to facilitate mobility in learning and working. Making qualifications and competences transparent is essential to promote mobility between European countries or within national sectors.

Open Badges can also offer an effective solution for numeracy (and other literacies).
Read more about Open Badges in Appendix 1 or go to www.openbadges.org

Conditions for development

The development of a certified numeracy  curriculum as described above requires brainpower, development and testing time, but above all mental space for new images of numerate  professionals who are ready for the 21st century.

Let’s avoid another thousand flowers having to bloom to perhaps get one flower out of them. That is why, on the basis of the above, we argue for:

– The further development of a widely supported vision document;
– outlining the frameworks within which the vision document can be worked out in practice and in cooperation;
– sketching a timetable in which the transformation of numeracy education (per ROC) takes place step by step;
– the establishment and facilitation of experimental groups with experienced members who can develop living labs and test them (or have them tested) on the basis of these frameworks.

– Consult Hogeschool Utrecht and Stichting PROMORE to share (international) knowledge.

For more information:
Kees Hoogland, University of Applied Sciences Utrecht, kees.hoogland@hu.nl
Ruben IJzerman, Stichting Promore, ruben@intraquest.nl

Kees Hoogland is senior lecturer in Didactics of Mathematics and Mathematics in Vocational Education at the Knowledge Centre for Learning and Innovation.

Ruben IJzerman is a developer of learning systems and innovative computing software and chairman of the PROMORE foundation (Professionalisering Modern Rekenonderwijs).

In een Open Badge zitten links ingebakken die aangeven wie de certificerende instantie is en welke bewijslast aan de badge gekoppeld is.

Some (still fictitious) practical examples of Open Badges Digitisation (and/or Functional Calculation)

Student 1 has already obtained a number of open badges on the basic level, namely numeracy – general at A1 level.
At the ROC he hopes to supplement this with the badges numeracy – general at the A2 level and numeracy – citizenship at the B1 level.
In addition, he sees that it is important for his chosen profession that he also obtains the badges numeracy-professional hairdresser at B1 level.

Student 2 has achieved on the blended vocational stream all basic numeracy badges and also an extra badge numeracy-professional-IT at B1 level. This gives her a head start in training to become an IT specialist. All she has to do is add two badges of numeracy to IT professionals at the B2 level.
She would also like to do the badges numeracy-citizen at B1 level, because working with numbers is her cup of tea and it will give her a head start in future applications as well.

Student 3 has always had major problems with mathematics. His parent did not have the money to buy a dyscalculia certificate, and since then he has avoided mathematics and numeracy as much as possible.
Now at the ROC he has been offered the opportunity to do the badge numeracy-development at A1 level, where he will try to see with a lot of guidance where his limits lie and which problems can be solved and which problems will be worked around. In the end he wants to be able to deal with money, rent and budgets independently.

Student 4 graduated last year and quickly found a job. In her personal development plan it was agreed that she would work partly at the workplace  and partly with guidance from the ROC on open badge numeracy-professional-communication at C1 level.